ANSWERS TO KNOT X.
§ 1. THE CHELSEA PENSIONERS.
Problem.---If 70 per cent. have lost an eye, 75 per cent. an ear, 80 per cent. an arm, 85 per cent. a leg: what percentage, at least, must have lost all four?
Answer.---Ten.
Solution.---(I adopt that of POLAR STAR, as being better than my own.) Adding the wounds together, we get 70 + 75 + 80 + 85 = 310, among 100 men; which gives 3 to each, and 4 to 10 men. Therefore the least percentage is 10.
Nineteen answers have been received. One is "5," but, as no working is given with it, it must, in accordance with the rule, remain "a deed without a name." JANET makes it "35 and 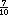 ths." I am sorry she has misunderstood the question, and has supposed that those who had lost an ear were 75 per cent. of those who had lost an eye; and so on. Of course, on this supposition, the percentages must all be multiplied together. This she has done correctly, but I can give her no honours, as I do not think the question will fairly bear her interpretation. THREE SCORE AND TEN makes it "19 and
ths." I am sorry she has misunderstood the question, and has supposed that those who had lost an ear were 75 per cent. of those who had lost an eye; and so on. Of course, on this supposition, the percentages must all be multiplied together. This she has done correctly, but I can give her no honours, as I do not think the question will fairly bear her interpretation. THREE SCORE AND TEN makes it "19 and 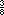 ths." Her solution has given me---I will not say "many anxious days and sleepless nights," for I wish to be strictly truthful, but---some trouble in making any sense of all of it. She makes the number of "pensionerswounded once" to be 310 ("pre cent.," I suppose!): dividing by 4, she gets 77 and a half as "average percentage:" again dividing by 4, she gets 10 and
ths." Her solution has given me---I will not say "many anxious days and sleepless nights," for I wish to be strictly truthful, but---some trouble in making any sense of all of it. She makes the number of "pensionerswounded once" to be 310 ("pre cent.," I suppose!): dividing by 4, she gets 77 and a half as "average percentage:" again dividing by 4, she gets 10 and 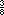 ths. as "percentage wounded four times." Does she suppose wounds of different kinds to "absorb" each other, so to speak? Then, no doubt, the data are equivalent to 77 pensioners with one wound each, and a half-pensioner with a half-wound. And does she then suppose these concentrated wounds to be transferable, so that
ths. as "percentage wounded four times." Does she suppose wounds of different kinds to "absorb" each other, so to speak? Then, no doubt, the data are equivalent to 77 pensioners with one wound each, and a half-pensioner with a half-wound. And does she then suppose these concentrated wounds to be transferable, so that 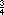 ths of these unfortunates can obtain perfect health by handing over their wounds to the remaining
ths of these unfortunates can obtain perfect health by handing over their wounds to the remaining 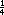 th? Granting these suppositions, her answer is right; or rather, if the question had been "A road is covered with one inch of gravel, along 77 and a half per cent. of it. How much of it ould be covered 4 inches deep with the same material?" her answer would have been right. But alas, that wasn't the question! DELTA makes some most amazing assumptions: "let every one who has not lost an eye have lost an ear," "let every one who has not lost both eyes and ears have lost an arm." Her ideas of a battle-field are grim indeed. Fancy a warrior who would continue fighting after losing both eyes, both ears, and both arms! This is a case which she (or "it?") evidently considers possible.
th? Granting these suppositions, her answer is right; or rather, if the question had been "A road is covered with one inch of gravel, along 77 and a half per cent. of it. How much of it ould be covered 4 inches deep with the same material?" her answer would have been right. But alas, that wasn't the question! DELTA makes some most amazing assumptions: "let every one who has not lost an eye have lost an ear," "let every one who has not lost both eyes and ears have lost an arm." Her ideas of a battle-field are grim indeed. Fancy a warrior who would continue fighting after losing both eyes, both ears, and both arms! This is a case which she (or "it?") evidently considers possible.
Next come eight writers who have made the unwarrantable assumption that, because 70 per cent. have lost an eye, therefore 30 per cent. have not lost one, so that they have both eyes. This is illogical. If you give me a bag of 100 sovereigns, and if in an hour I come back to you (my face not beaming with gratitude nearly so much as when I received the bag) to say "I am sorry to tell you that 70 of these sovereigns are bad," do I thereby guarantee the other 30 to be good? Perhaps I have not tested them yet. The sides of this illogical octagon are as follows, in alphabetical order:---ALGERNON BRAY, DINAH MITE, G. S. C., JANE E., J. D. W., MAGPIE (who makes the delightful remark "therefore 90 per cent. have two of something," recalling to one's memory that fortunate monarch, with whom Xerxes was so much pleased that "he gave him ten of everything!"), S. S. G., and TOKIO.
BRADSHAW OF THE FUTURE and T. R. do the question in a piecemeal fashion---on the principle that the 70 per cent. and the 75 per cent., though commenced at opposite ends of the 100, must overlap by at least 45 per cent.; and so on. This is quite correct working, but not, I think, quite the best way of doing it.
The other five competitors will, I hope, feel themselves sufficiently glorified by placed in the first class, without my composing a Triumphal Ode for each!
CLASS LIST.
I.
| OLD CAT. |
 |
POLAR STAR. |
OLD HEN.
 |
SIMPLE SUSAN. |
| WHITE SUGAR. |
 |
|
II.
| BRADSHAW OF THE FUTURE. |
 |
T. R. |
III.
| ALGERNON BRAY. |
 |
J. D. W. |
| DINAH MITE. |
 |
MAGPIE. |
| G. S. C. |
 |
S. S. G. |
| JANE E. |
 |
TOKIO. |
§ 2. CHANGE OF DAY.
I must postpone, sine die, the geographical problem-partly because I have not yet received the statistics I am hoping for, and partly because I am myself so entirely puzzled by it; and when an examiner is himself dimly hovering between a second class and a third how is he to decide the position of others?
§ 3. THE SONS' AGES.
Problem.---"At first, two of the ages are together equal to the third. A few years afterwards, two of them are together double of the third. When the number of years since the first occasion is two-thirds of the sum of the ages on that occasion, one age is 21. What are the other two?"
Answer.---"15 and 18"
Solution.-Let the ages at first be x, y, (x+y). Now if a+b = 2c, then (a-n) + (b-n) = 2(c-n), whatever be the value of of n. Hence the second relationship, if ever true, was always true. Hence it was true at first. But it cannot be that x and y are together double of (x+ y). Hence it must be true of (x+ y) together with x or y; and it does not matter which we take. We assume, then, (x+ y) + x = 2y, i.e. y = 2x. Hence the three ages were, at first, x, 2x, 3x; and the number of years, since that time is two-thirds of 6x, i.e. is 4x. Hence the present ages are 5x, 6x, 7x. The ages are clearly integers, since this is only "the year when one of my sons comes of age." Hence 7x=21, x=3, and the other ages are 15, 18.
Eighteen answers have been received. One of the writers merely asserts that the first occasion was 12 years ago, that the ages were then 9, 6, and 3; and that on the second occasion they were 14, 11, and 8! As a Roman father, I ought to withhold the name of the rash writer; but respect for age makes me break the rule: it is THREE AND TEN. JANE E. also asserts that the ages at first were 9, 6, 3: then she calculates the present ages, leaving the second occasion unnoticed. OLD HEN is nearly as bad; she "tried various numbers till I found one that fitted all the conditions"; but merely scratching up the earth, and pecking about, is not the way to solve a problem, oh venerable bird! And close after OLD HEN prowls, with hungry eyes, OLD CAT, who calmly assumes, to begin with, that the son who comes of age is the eldest. Eat your bird, Puss, for you will get nothing from me!
There are yet two zeroes to dispose of. MINERVA assumes that, on every occasion, a son comes of age; and that it is only such a son who is "tipped with gold." Is it wise thus to interpret "now, my boys, calculate your ages, and you shall have the money"? BRADSHAW OF THE FUTURE says "let" the ages at first be 9, 6, 3, then assumes the second occasion was 6 years afterwards, and on these baseless assumptions brings out the right answers. Guide future travellers, an thou wilt: thou art no Bradshaw for this Age!
Of those who win honours, the merely "honourable" are two. DINAH MITE ascertains (rightly) the relationship between the three ages at first, but then assumes one of them to be "6," thus making the rest of her solution tentative. M. F. C. does the algebra all right up to the conclusion that the present ages are 5z, 6z, and 7z; it then assumes, without giving any reason, that 7z = 21.
Of the more honourable, DELTA attempts a novelty---to discover which son comes of age by elimination: it assumes, successively, that it is the middle one, and that it is the youngest; and in each case it apparently brings out an absurdity. Still, as the proof contains the following bit of algebra, "63 = 7x + 4y;  21 = x + 4 sevenths of y," I trust it will admit that its proof is not quite conclusive. The rest of its work is good. MAGPIE betrays the tendency of the tribe---to appropriate any stray conclusion she comes across, without having any strict logical right to it. Assuming A, B, C, as the ages at first, and D as the number of years that have elapsed since then, she finds (rightly) the 3 equations, 2A = B, C = B + A, D = 2B. She then says "supposing that A = 1, then B = 2, C = 3, and D = 4. Therefore for A, B, C, D, four numbers are wanted which shall be to each other as 1 : 2 : 3 : 4." It is in the "therefore" that I detect the unconscientiousness of this bird. The conclusion is true, but this is only because the equations are "homogeneous" (i.e. having one "unknown" in each term), a fact which I strongly suspect had not been grasped---I beg pardon, clawed---by her. Were I to lay this little pitfall, "A + 1 = B, B + 1 = C; supposing A = 1, then B = 2, and C = 3. Therefore for A, B, C, three numbers are wanted which shall be to one another as 1 : 2 : 3," would you not flutter down into it, oh MAGPIE, as amiably as a Dove? SIMPLE SUSAN is anything but simple to me. After ascertaining that the 3 ages at first are as 3 : 2 : 1, she says "then, as two-thirds of their sum, added to one of them, = 21, the sum cannot exceed 30, and consequently the highest cannot exceed 15." I suppose her (mental) argument is something like this:---"two-thirds of sum, + one age, = 21;
21 = x + 4 sevenths of y," I trust it will admit that its proof is not quite conclusive. The rest of its work is good. MAGPIE betrays the tendency of the tribe---to appropriate any stray conclusion she comes across, without having any strict logical right to it. Assuming A, B, C, as the ages at first, and D as the number of years that have elapsed since then, she finds (rightly) the 3 equations, 2A = B, C = B + A, D = 2B. She then says "supposing that A = 1, then B = 2, C = 3, and D = 4. Therefore for A, B, C, D, four numbers are wanted which shall be to each other as 1 : 2 : 3 : 4." It is in the "therefore" that I detect the unconscientiousness of this bird. The conclusion is true, but this is only because the equations are "homogeneous" (i.e. having one "unknown" in each term), a fact which I strongly suspect had not been grasped---I beg pardon, clawed---by her. Were I to lay this little pitfall, "A + 1 = B, B + 1 = C; supposing A = 1, then B = 2, and C = 3. Therefore for A, B, C, three numbers are wanted which shall be to one another as 1 : 2 : 3," would you not flutter down into it, oh MAGPIE, as amiably as a Dove? SIMPLE SUSAN is anything but simple to me. After ascertaining that the 3 ages at first are as 3 : 2 : 1, she says "then, as two-thirds of their sum, added to one of them, = 21, the sum cannot exceed 30, and consequently the highest cannot exceed 15." I suppose her (mental) argument is something like this:---"two-thirds of sum, + one age, = 21;  sum, + 3 halves one age, = 31 and a half. But 3 halves of one age cannot be less than 1 and-a-half (here I perceive that SIMPLE SUSAN would on no account present a guinea to a new-born baby!) hence the sum cannot exceed 30." This is ingenious, but her proof, after that is (as she candidly admits) "clumsy and roundabout." She finds that there are 5 possible sets of ages, and eliminates four of them. Suppose that, instead of 5, there ahd been 5 million possible sets? Would SIMPLE SUSAN have courageously ordered in the necessary gallon of ink and ream of paper?
sum, + 3 halves one age, = 31 and a half. But 3 halves of one age cannot be less than 1 and-a-half (here I perceive that SIMPLE SUSAN would on no account present a guinea to a new-born baby!) hence the sum cannot exceed 30." This is ingenious, but her proof, after that is (as she candidly admits) "clumsy and roundabout." She finds that there are 5 possible sets of ages, and eliminates four of them. Suppose that, instead of 5, there ahd been 5 million possible sets? Would SIMPLE SUSAN have courageously ordered in the necessary gallon of ink and ream of paper?
The solution sent in by C.R. is, like that of SIMPLE SUSAN, partly tentative, and so does not rise higher than being Clumsily Right.
Among those who have earned the highest honours, ALGERNON BRAY solves the problem quite correctly, but adds that there is nothing to exclude the supposition that all the ages were fractional. This would make the number of answers infinite. Let me meekly protest that I never intended my readers to devote the rest of their lives to writing out answers! E. M. RIX points out that, if fractional ages be admissible, any one of the three sons might be the one "come of age" but she rightly rejects this supposition on the ground that it would make the problem indeterminate. WHITE SUGAR is the only one who has detected an oversight of mine: I had forgotten the possibility (which of course ought to be allowed for) that the son, who came of age that year, need not have done so by that day, so that he might be only 20. This gives a second solution, viz., 20, 24, 28. Well said, pure Crystal! Verily, thy "fair discourse has been as sweet as sugar"!
CLASS LIST.
I.
| ALGERNON BRAY. |
 |
S. S. G. |
| AN OLD FOGEY. |
 |
TOKIO. |
| E. M. RIX. |
 |
T. R. |
| G. S. C. |
 |
WHITE SUGAR. |
II.
| C. R. |
 |
MAGPIE. |
| DELTA. |
 |
SIMPLE SUSAN. |
III.
| DINAH MITE. |
 |
M. F. C. |
I have received more than one remonstrance on my assertion, in the Chelsea Pensioners' problem, that it was illogical to assume, from the datum "70 p. c. have lost an eye," that 30 p. c. have not. ALGERNON BRAY states, as a parallel case, "suppose Tommy's father gives him 4 apples, and he eats one of them, how many has he left?" and says, "I think we are justified in answering, 3." I think so too. There is no "must" here, and the data are evidently meant to fix the answer exactly: but if the question were set me "how many must he have left?", I should understand the data to be that his father gave him 4 at least, but may have given him more.
I take this opportunity of thanking those who have sent, along with their answers to the Tenth Knot, regrets that there are no more Knots to come, or petitions that I should recall my resolution to bring them to an end. I am most grateful for their kind words; but I think it wisest to end what, at best, was but a lame attempt. "The stretched metre of an antique song" is beyond my compass; and my puppets were neither distinctly in my life (like those I now address), nor yet (like Alice and the Mock Turtle) distinctly out of it. Yet let me at least fancy, as I lay down my pen, that I carry with me into my silent life, dear reader, a farewell smile from your unseen face, and a kindly farewell pressure from your unfelt hand! And so, good night! Parting is such sweet sorrow, that I shall say "good night!" till it be morrow.
THE END
Stefan Bilaniuk,
Department of Mathematics,
Trent University
Maintained by Stefan Bilaniuk.
Last updated 1998.08.22.
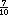 ths." I am sorry she has misunderstood the question, and has supposed that those who had lost an ear were 75 per cent. of those who had lost an eye; and so on. Of course, on this supposition, the percentages must all be multiplied together. This she has done correctly, but I can give her no honours, as I do not think the question will fairly bear her interpretation. THREE SCORE AND TEN makes it "19 and
ths." I am sorry she has misunderstood the question, and has supposed that those who had lost an ear were 75 per cent. of those who had lost an eye; and so on. Of course, on this supposition, the percentages must all be multiplied together. This she has done correctly, but I can give her no honours, as I do not think the question will fairly bear her interpretation. THREE SCORE AND TEN makes it "19 and 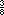 ths." Her solution has given me---I will not say "many anxious days and sleepless nights," for I wish to be strictly truthful, but---some trouble in making any sense of all of it. She makes the number of "pensionerswounded once" to be 310 ("pre cent.," I suppose!): dividing by 4, she gets 77 and a half as "average percentage:" again dividing by 4, she gets 10 and
ths." Her solution has given me---I will not say "many anxious days and sleepless nights," for I wish to be strictly truthful, but---some trouble in making any sense of all of it. She makes the number of "pensionerswounded once" to be 310 ("pre cent.," I suppose!): dividing by 4, she gets 77 and a half as "average percentage:" again dividing by 4, she gets 10 and 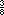 ths. as "percentage wounded four times." Does she suppose wounds of different kinds to "absorb" each other, so to speak? Then, no doubt, the data are equivalent to 77 pensioners with one wound each, and a half-pensioner with a half-wound. And does she then suppose these concentrated wounds to be transferable, so that
ths. as "percentage wounded four times." Does she suppose wounds of different kinds to "absorb" each other, so to speak? Then, no doubt, the data are equivalent to 77 pensioners with one wound each, and a half-pensioner with a half-wound. And does she then suppose these concentrated wounds to be transferable, so that 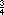 ths of these unfortunates can obtain perfect health by handing over their wounds to the remaining
ths of these unfortunates can obtain perfect health by handing over their wounds to the remaining 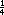 th? Granting these suppositions, her answer is right; or rather, if the question had been "A road is covered with one inch of gravel, along 77 and a half per cent. of it. How much of it ould be covered 4 inches deep with the same material?" her answer would have been right. But alas, that wasn't the question! DELTA makes some most amazing assumptions: "let every one who has not lost an eye have lost an ear," "let every one who has not lost both eyes and ears have lost an arm." Her ideas of a battle-field are grim indeed. Fancy a warrior who would continue fighting after losing both eyes, both ears, and both arms! This is a case which she (or "it?") evidently considers possible.
th? Granting these suppositions, her answer is right; or rather, if the question had been "A road is covered with one inch of gravel, along 77 and a half per cent. of it. How much of it ould be covered 4 inches deep with the same material?" her answer would have been right. But alas, that wasn't the question! DELTA makes some most amazing assumptions: "let every one who has not lost an eye have lost an ear," "let every one who has not lost both eyes and ears have lost an arm." Her ideas of a battle-field are grim indeed. Fancy a warrior who would continue fighting after losing both eyes, both ears, and both arms! This is a case which she (or "it?") evidently considers possible. 21 = x + 4 sevenths of y," I trust it will admit that its proof is not quite conclusive. The rest of its work is good. MAGPIE betrays the tendency of the tribe---to appropriate any stray conclusion she comes across, without having any strict logical right to it. Assuming A, B, C, as the ages at first, and D as the number of years that have elapsed since then, she finds (rightly) the 3 equations, 2A = B, C = B + A, D = 2B. She then says "supposing that A = 1, then B = 2, C = 3, and D = 4. Therefore for A, B, C, D, four numbers are wanted which shall be to each other as 1 : 2 : 3 : 4." It is in the "therefore" that I detect the unconscientiousness of this bird. The conclusion is true, but this is only because the equations are "homogeneous" (i.e. having one "unknown" in each term), a fact which I strongly suspect had not been grasped---I beg pardon, clawed---by her. Were I to lay this little pitfall, "A + 1 = B, B + 1 = C; supposing A = 1, then B = 2, and C = 3. Therefore for A, B, C, three numbers are wanted which shall be to one another as 1 : 2 : 3," would you not flutter down into it, oh MAGPIE, as amiably as a Dove? SIMPLE SUSAN is anything but simple to me. After ascertaining that the 3 ages at first are as 3 : 2 : 1, she says "then, as two-thirds of their sum, added to one of them, = 21, the sum cannot exceed 30, and consequently the highest cannot exceed 15." I suppose her (mental) argument is something like this:---"two-thirds of sum, + one age, = 21;
21 = x + 4 sevenths of y," I trust it will admit that its proof is not quite conclusive. The rest of its work is good. MAGPIE betrays the tendency of the tribe---to appropriate any stray conclusion she comes across, without having any strict logical right to it. Assuming A, B, C, as the ages at first, and D as the number of years that have elapsed since then, she finds (rightly) the 3 equations, 2A = B, C = B + A, D = 2B. She then says "supposing that A = 1, then B = 2, C = 3, and D = 4. Therefore for A, B, C, D, four numbers are wanted which shall be to each other as 1 : 2 : 3 : 4." It is in the "therefore" that I detect the unconscientiousness of this bird. The conclusion is true, but this is only because the equations are "homogeneous" (i.e. having one "unknown" in each term), a fact which I strongly suspect had not been grasped---I beg pardon, clawed---by her. Were I to lay this little pitfall, "A + 1 = B, B + 1 = C; supposing A = 1, then B = 2, and C = 3. Therefore for A, B, C, three numbers are wanted which shall be to one another as 1 : 2 : 3," would you not flutter down into it, oh MAGPIE, as amiably as a Dove? SIMPLE SUSAN is anything but simple to me. After ascertaining that the 3 ages at first are as 3 : 2 : 1, she says "then, as two-thirds of their sum, added to one of them, = 21, the sum cannot exceed 30, and consequently the highest cannot exceed 15." I suppose her (mental) argument is something like this:---"two-thirds of sum, + one age, = 21;