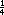 minutes.
minutes.Answer.---Place 8 pigs in the first sty, 10 in the second, nothing in the third, and 6 in the fourth: 10 is nearer ten than 8; nothing is nearer ten than 10; 6 is nearer ten than nothing; and 8 is nearer ten than 6.
This problem is noticed by only two correspondents. BALBUS says "it certainly cannot be solved mathematically, nor do I see how to solve it by any verbal quibble." NOLENS VOLENS makes Her Radiancy change the direction of going round; and even then is obliged to add "the pigs must be carried in front of her"!
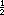 minutes: when will he be overtaken by one?
minutes: when will he be overtaken by one?
Answer.---In 6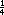 minutes.
minutes.
Solution.---Let "a" be the distance an omnibus goes in 15 minutes, and "x" the distance from the starting-point to where the traveller is overtaken. Since the omnibus met is due at the starting-point in 2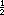 minutes, it goes in that time as far as the traveller walks in 12
minutes, it goes in that time as far as the traveller walks in 12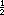 ; i.e. it goes 5 times as fast. Now the overtaking omnibus is "a" behind the traveller when he starts, and therefore goes "a+x" while he goes "x." Hence a+x = 5x; i.e. 4x = a, and x = a/4. This distance would be traversed by an omnibus in 15/4 minutes, and therefore by the traveller in 5 * 15/4. Hence he is overtaken in 18
; i.e. it goes 5 times as fast. Now the overtaking omnibus is "a" behind the traveller when he starts, and therefore goes "a+x" while he goes "x." Hence a+x = 5x; i.e. 4x = a, and x = a/4. This distance would be traversed by an omnibus in 15/4 minutes, and therefore by the traveller in 5 * 15/4. Hence he is overtaken in 18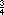 minutes after starting, i.e. in 6
minutes after starting, i.e. in 6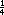 minutes after meeting the omnibus.
minutes after meeting the omnibus.
Four answers have been received, of which two are wrong. DINAH MITE rightly states that the overtaking omnibus reached the point where they met the other omnibus 5 minutes after they left, but wrongly concludes that, going 5 times as fast, it would overtake them in another minute. The travellers are 5-minutes-walk ahead of the omnibus, and must walk 1-4th of this distance farther before the omnibus overtakes them, which will be 1-5th of the distance traversed by the omnibus in the same time: this will require 1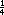 minutes more. NOLENS VOLENS tries it by a process like "Achilles and the Tortoise." He rightly states that, when the overtaking omnibus leaves the gate, the travellers are 1-5th of "a" ahead, and that it will take the omnibus 3 minutes to traverse this distance; "during which time" the travellers, he tells us, go 1-15th of "a" (this should be 1-25th). The travellers being now 1-15th of "a" ahead, he concludes that the work remaining to be done is for the travellers to go 1-60th of "a," while the omnibus goes 1-12th. The principle is correct, and might have been applied earlier.
minutes more. NOLENS VOLENS tries it by a process like "Achilles and the Tortoise." He rightly states that, when the overtaking omnibus leaves the gate, the travellers are 1-5th of "a" ahead, and that it will take the omnibus 3 minutes to traverse this distance; "during which time" the travellers, he tells us, go 1-15th of "a" (this should be 1-25th). The travellers being now 1-15th of "a" ahead, he concludes that the work remaining to be done is for the travellers to go 1-60th of "a," while the omnibus goes 1-12th. The principle is correct, and might have been applied earlier.
| BALBUS. | DELTA. |
| NEXT | Title | Epigraph | Preface | Contents |
| Knot | I II III IV V VI VII VIII IX X |
| Answers | I II III IV V VI VII VIII IX X |